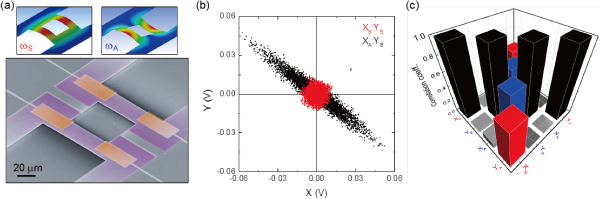
The widespread availability of quantum entanglement with photons, in the guise of two-mode squeezed states, can be attributed to the phenomenon of parametric down-conversion. A reinterpretation of this effect with macroscopic mechanical objects can offer a route towards a purely mechanical entanglement and the unique possibility of probing the quantum mechanical nature of our everyday classical world. In spite of this prospect, mechanical two-mode squeezed states have remained elusive due to the inability to recreate the nonlinear interaction at the heart of this phenomenon in the mechanical domain.
To address this we have developed a non-degenerate parametric down-converter, in a mechanical resonator integrated with electrical functionality, which enables mechanical nonlinearities to be dynamically engineered to emulate this interaction [1]. In this configuration, phonons are simultaneously generated in pairs in two macroscopic vibration modes which results in the amplification of their motion by more than 20 dB. In parallel, mechanical two-mode squeezed states are also created which exhibit fluctuations 5 dB below the thermal level of their constituent modes as well as harbouring correlations between the modes that become almost perfect as their amplification is increased. This remarkable observation of correlations between two massive phonon ensembles paves the way towards an entangled macroscopic mechanical system at the single phonon level.
This work was supported by KAKENHI