Atsushi Ishizawa and Hidetoshi Nakano
Optical Science Laboratory
The carrier-envelope phase (CEP) is the phase of the carrier wave at the maximum of the envelope. The timing of the oscillation cycles within a light pulse plays a role in the interaction of light with matter when the duration of the pulse becomes comparable to the light oscillation period. Therefore, it is necessary to control the CEP of few-cycle laser pulses in order to apply nonlinear spectroscopy.
To date, it has been observed that high-order harmonic generation [1]
and above-threshold ionization are sensitive to the CEP. Almost all previous
direct measurements of the CEP have relied on strong-field processes using
100-µJ amplified laser pulses, which are available only at low repetition
rates in a vacuum chamber equipped with a specialized detector. Therefore,
only a few laboratories have been able to measure the CEP.
We demonstrated a measurement that provides direct information about
the CEP of a few-cycle laser pulse. In any f -to-2f or 2 f -to-3f spectrum
interferogram method using a nonlinear crystal, there is a certain unknown
phase shift in the measured value because of the linear dispersion in the
SHG crystal. We have devised a way of directly measuring the CEP using
the interference between the second and third harmonics from the surface
of a solid. Both harmonics have a π/2 phase shift relative to the
fundamental optical field when they are generated from the surface of a
solid. This means the interference signals do not include a constant offset
phase component and can therefore be used to measure the CEP directly.
This method uses pulses with a low energy of less than 1 µJ, and
it’s very easy to set up the equipment and perform the measurement in
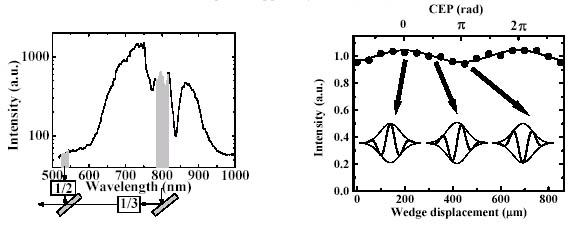
air. The spectrum of the laser pulse is shown in Fig. 1. In this experiment,
a 5-fs 1-µJ laser pulse was focused on a glass target at the incident
angle of 45°. Even and odd harmonics were generated due to the broken
symmetry of an interface. The second and third harmonic spectrums overlap
in the ultra-violet region. Figure 2 shows a series of interference signals
of different phases. The signals cyclically move up and down with the CEP,
which has a period of 2π rad. We will be able to achieve stabilization
of the CEP of a few-cycle amplified laser pulse by detecting it using this
method.
[1] A. Baltuska, et al., Nature. 421 (2003) 611.
[2] A. Ishizawa and H. Nakano, Jpn. J. Appl. Phys. 45 (2006) 4087.
 |
||||||
|
|
|||||