the Thickness of an Electric Double Layer in a Nanogap
Materials Science Laboratory
We have reported that the self-spreading of an artificial
cell membrane (ACM) on a solid support could be controlled electrostatically
by modulating an electric field applied between nanogap electrodes, where
we concluded that the electric double layer played a significant role in
the trap of the ACM [1]. In this study, we describe the mechanism of the
electrostatic control of ACM self-spreading, and investigate the dependence
of the ionic concentration of an electrolyte and the nanogap spacing under
finely controlled conditions [2].
A lipid mixture (molar ratio 7:3) consisting of uncharged L-α-phosphatidylcholine and negatively charged L-α-phosphatidylglycerol containing 1 mol% Texas Red-DHPE was prepared. A small amount of the solid was attached to the device. The self-spreading of an ACM was initiated by immersing the device in an electrolyte solution including 1-100 mM NaCl. A DC voltage (-50 mV) was applied between nanogap electrodes during operation.
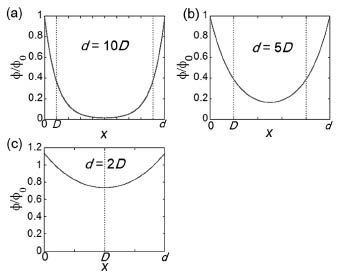
We calculated the electric potentials in the nanogap
as a function of the distance from one side of the electrode surfaces as
shown in Fig. 1. When the nanogap spacing (d) is sufficiently larger than the thickness of the electric double layer
(D), the electric field is shielded by counterions in an electrolyte solution leading to no voltage-dependent change in self-spreading [Fig. 1(a)]. In contrast, when d < 5D, the electric field can be effectively applied in the nanogap spacing,
leading to the electrostatic trapping of the ACM [Fig. 1(b, c)]. We performed
experiments to determine whether ACM self-spreading could be controlled
at each ionic concentration of NaCl (c) as a function of nanogap spacing.
For NaCl solutions, the thickness of the electric double layer is expressed
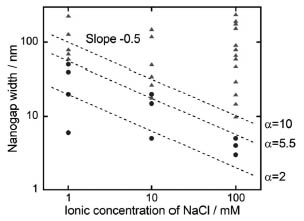
as D ~ 0.304 /
. The results are summarized in Fig. 2. The circles and triangles, respectively, show cases where self-spreading could and could not be controlled by applying a voltage. This result is suggestive of a certain threshold for controlling the self-spreading. Therefore, the maximum nanogap width for controlling the self-spreading at a certain ionic concentration is given by dmax ~αD ~ 0.304α /, where α is the threshold factor. According to the above equation, dotted
lines with a slope of -0.5 were drawn in Fig. 2 for each α value and the
line for α = 5.5 provided the best fit with the experiments. This is in
good agreement with the prediction obtained from the electric potential
calculation, where d = 5D is a threshold for the control of ACM self-spreading, suggesting the validity
of our proposed mechanism.
[1] Y. Kashimura et al., J. Am. Chem. Soc. 133 (2011) 6118.
[2] Y. Kashimura et al., IEICE TRANS. ELECTRON 96-C (2013) 344.
 |
 |
|||||
|
|